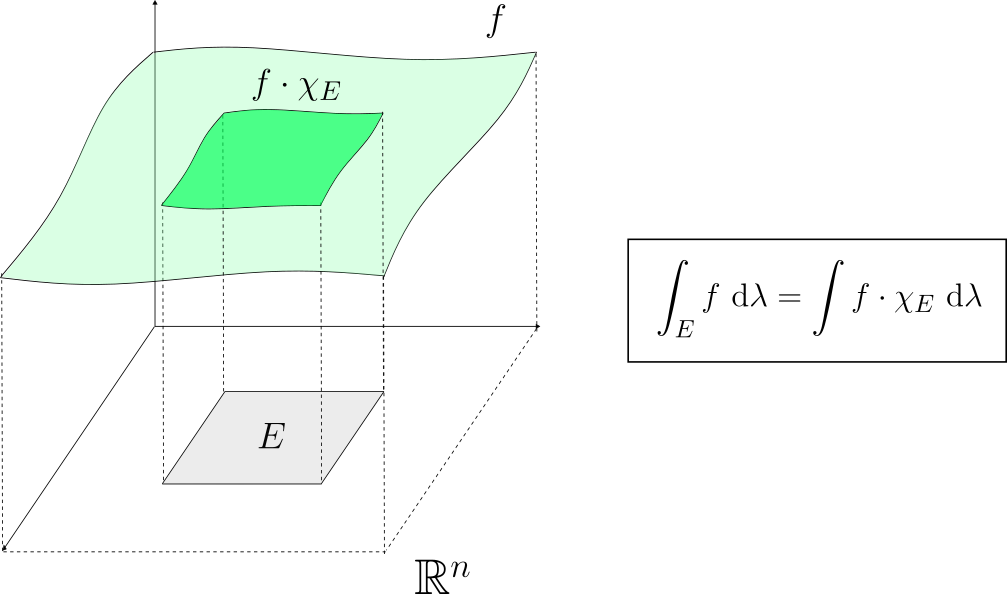
MATERIAL EN REVISIÓN
Introducción
El concepto de «casi donde sea» refiere a que, en términos de integración, dos objetos son en cierto modo idénticos si «coinciden salvo en conjuntos de medida cero».
Algunos Lemas
La siguiente serie de Lemas refuerzan la idea de que, a ojos de la integral, dos objetos son «iguales» si coinciden salvo en conjuntos de medida cero.
Lema. Sea una función medible. Si es una función tal que es un conjunto de medida cero, entonces es medible.
Demostración. Para cualquier , podemos escribir:
Donde Y
Claramente , así que y son medibles. (Reuerda que cualquier conjunto con medida exterior cero es Lebesgue medible). Como , y son medibles, se sigue entonces que es un conjunto medible.
Lema. Si es una función no negativa tal que salvo un conjunto de medida cero, entonces
Demostración. Por el Lema anterior, se sigue que es medible. Al ser no negativa, .
Si , por definición de la integral, existiría una función simple tal que y Al ser simple, podemos escribir , con y ajenos. Como , necesariamente existiría algún con . Sin embargo, esto implica que sobre , lo que contradice que salvo en un conjunto de medida cero.
Por tanto, la única posibilidad es .
De hecho, podemos relajar la condición en el Lema anterior:
Corolario. Si es una función tal que salvo un conjunto de medida cero, entonces y
Demostración. es medible por el primer Lema. Aplicando el lema anterior a y por separado, se sigue que
Proposición (Insensibilidad de la integral).
- Sea una función medible no negativa. Si es tal que el conjunto es de medida cero, entonces es medible y
- Sea una función en . Si es tal que el conjunto es de medida cero, entonces y
Demostración. Los lemas anteriores aseguran que es medible en ambos casos.
Para la primera parte, supongamos primero que es no negativa. Podemos escribir y , en ambos casos, los sumandos derecho son 0 salvo en conjuntos de medida nula, luego:
Para el caso general, podemos escribir , donde y salvo en conjuntos de medida cero. Usando el caso anterior y los lemas:
Para el segundo caso, notemos que salvo un conjunto de medida cero, de donde con
Luego, por linealidad, y
El concepto de casi donde sea
Definición. Decimos que una propiedad en se satisface «casi donde sea» ó «para casi todo » ó «para casi todo punto» si el conjunto
Es nulo (de medida de Lebesgue cero).
Generalmente abreviaremos la expresión «en casi todo punto» y sus equivalentes como c.t.p. En la literatura inglesa se suele denotar como a.e. (por almost everywhere).
Ejemplo. Casi todos los números reales son irracionales, pues el conjunto de racionales es de medida cero ( es numerable).
Ejemplo. Podemos reescribir la proposición de insensibilidad de la siguiente forma: Si y en c.t.p entonces y . De modo que, a ojos de la integral, dos funciones iguales en c.t.p. son «indistinguibles».
Otra situación que ocurre con frecuencia es que sólo podamos asegurar que una función esté definida en c.t.p (el último teorema de esta sección es un ejemplo de esto). Sin embargo, como sugieren los teoremas anteriores, esto es suficiente para poder hablar de su integral. Procedamos de manera precisa.
Definición. Sea una función definida en c.t.p de . Decimos que es medible si existe una extensión medible de , digamos . Definimos la integral de como (Siempre que ésta tenga sentido).
Observación. Hay que notar que si admite una extensión medible, entonces cualquier extensión de será medible (primer lema de la entrada), además, la integral no depende de la extensión tomada (insensibilidad). Por esta razón, típicamente tomamos la «extensión por cero», es decir, la extensión que vale cero en los puntos donde no está definida originalmente.
Generalización de los Teoremas de convergencia
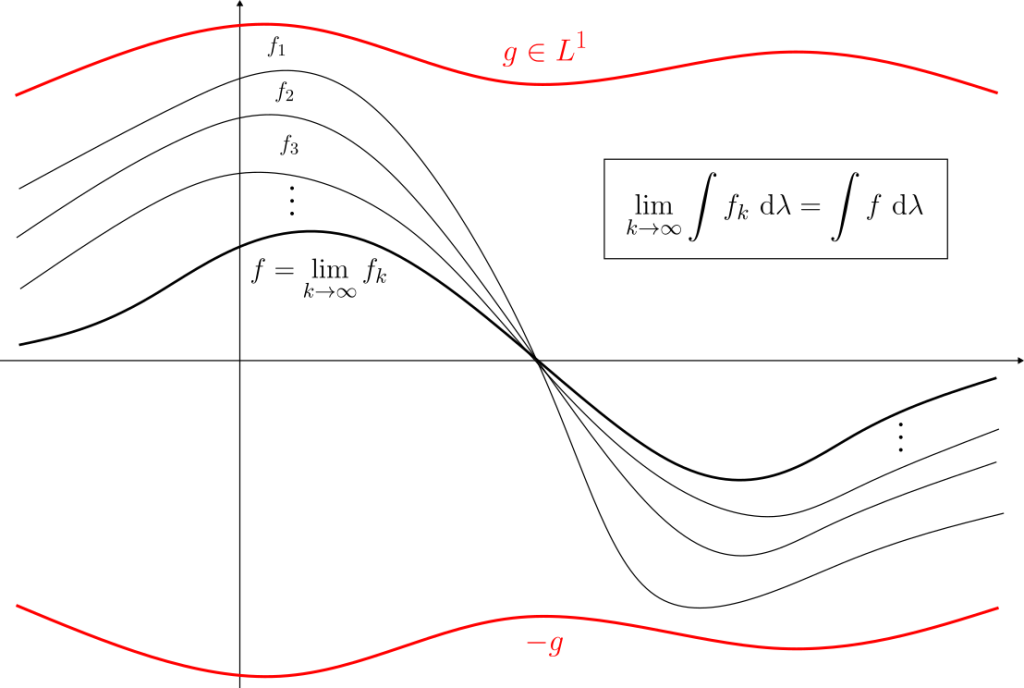
Podemos dar generalizaciones de los teoremas de convergencia en las que consideremos propiedades en casi todo punto. Por ejemplo, para el teorema de convergencia dominada tendríamos lo siguiente.
Proposición (Convergencia dominada versión c.t.p.) Sean funciones medibles definidas en c.t.p. de , tales que
Existe para casi todo y además existe una función definida en c.t.p con
Para casi todo . Entonces
Demostración. Sea el conjunto de tales que: O bien no está definida para algún , o bien no existe, o bien para algún . Como podemos expresar a como una unión numerable de conjuntos nulos (¿Cuáles?), el propio debe ser nulo.
Podemos redefinir las y de tal manera que valgan 0 sobre (esto no afecta las propiedades en c.t.p. ni los valores de ninguna integral). El resultado se sigue de aplicar el teorema de la convergencia dominada usual sobre estas nuevas funciones y luego apelar a la insensibilidad de la integral.
Podemos dar generalizaciones similares para los teoremas de convergencia monótona y el Lema de Fatou. A partir de ahora no haremos distinción entre las versiones usuales y en c.t.p. de estos teoremas.
Intercambio de Sumas con Integrales
El siguiente resultado es relevante. Como adelantamos, es un ejemplo en el que sólo podemos asegurar que una función esté definida en c.t.p.
Teorema. Sean funciones en , tales que Entonces Existe para casi todo y además
Demostración. Sea . Al ser una suma de funciones positivas, ya habíamos probado (como consecuencia del teorema de la convergencia monótona) que:
Al ser una función en , sabemos que en c.t.p. la serie converge absolutamente para casi todo , por lo que en particular converge para casi todo . Además, es claro que: para casi todo . Aplicando el teorema de la convergencia dominada sobre la sucesión de sumas parciales concluimos:
Más adelante…
Definiremos la integral sobre subconjuntos de y sus principales propiedades.
Tarea moral