MATERIAL EN REVISIÓN

Introducción

En esta entrada definiremos la clase de conjuntos de Borel $\mathcal{B}$, que será junto a la clase de conjuntos Lebesgue medibles $\mathcal{L}$, esencial para construir la integral de Lebesgue en el futuro.

Conjuntos de Borel

Definición. La clase de conjuntos de Borel ${\mathcal{B}}_n$ en ${\mathbb{R}}^n$ es la $\sigma$-álgebra generada [ENLACE] por la colección de conjuntos abiertos. Cuando la dimensión sea clara del contexto, denotaremos a los conjuntos de Borel simplemente como $\mathcal{B}$.

Observación. $\mathcal{B}$ contiene a todos los conjuntos abiertos y cerrados.

Observación. Como ya probamos, la clase de conjuntos Lebesgue medibles es una $\sigma$-álgebra que contiene a los abiertos, de modo que: $\mathcal{B}\subseteq \mathcal{L}$ (definición de $\sigma$-álgebra generada). De hecho, es posible probar que la contención es estricta (aunque lo omitiremos). Puedes consultar un contraejemplo clásico en [ENLACE].

Los conjuntos de Borel se pueden pensar como «la $\sigma$-álgebra que tiene mejor relación con la topología de ${\mathbb{R}}^n$». Más adelante esto será crucial para construir una noción de integración que tenga una buena relación con límites y convergencias.

Algunas propiedades de los conjuntos de Borel

A pesar de no ser iguales, la clase de conjuntos de Borel $\mathcal{B}$ es «casi» la clase de conjuntos Lebesgue medibles $\mathcal{L}$ como muestra el siguiente teorema.

Teorema. Si $A\subseteq {\mathbb{R}}^n$ es Lebesgue medible, entonces admite una descomposición de la forma $$A=E\cup N.$$ Donde $E\cap N=\mathrm{\varnothing }$, $E$ es un conjunto de Borel y $N$ es nulo.

Demostración. Por las equivalencias de conjuntos medibles, para cada $k\in \mathbb{N}$, podemos encontrar un cerrado $F_k\subseteq A$ tal que $$\lambda (A\setminus F_k)<\frac{1}{k}.$$ Definamos $E=\bigcup _{k=1}^{\mathrm{\infty }}{F}_k$. Claramente $E\subseteq A$ y $E\in \mathcal{B}$ (es unión numerable de conjuntos de Borel). Ahora, para cualquier $k\in \mathbb{N}$: $$\lambda (A\setminus E)\le \lambda (A\setminus F_k)<\frac{1}{k}$$ $$⟹\lambda (A\setminus E)=0.$$ Así que una posible descomposición es: $$N:=E\cup (A\setminus E).$$

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Conjuntos de Borel y funciones continuas

Como los conjuntos de Borel están definidos en «términos topológicos», es de esperarse que tengan una relación fuerte con las funciones continuas.

Teorema. Sea $E$ un conjunto de Borel en ${\mathbb{R}}^n$. Sea $f:E\to {\mathbb{R}}^m$ una función continua. Si $A\in {\mathcal{B}}_m$ , entonces $f^{-1}(A)\in {\mathcal{B}}_n$.

Demostración. La idea es explotar la estructura de $\sigma$-álgebra (tanto en ${\mathbb{R}}^n$ como en ${\mathbb{R}}^m$) para probar la proposición. Definamos: $$\mathcal{M}=\{A|A\subseteq {\mathbb{R}}^m\text{ y }{f}^{-1}(A)\in {\mathcal{B}}_n\}.$$

Entonces, necesitamos probar que ${\mathcal{B}}_m\subseteq \mathcal{M}$. Como ${\mathcal{B}}_m$ es la $\sigma$-álgebra más pequeña que contiene a los abiertos de ${\mathbb{R}}^m$, basta probar que $\mathcal{M}$ es una $\sigma$-álgebra que contiene a todos los abiertos de ${\mathbb{R}}^m$ para que ${\mathcal{B}}_m\subseteq \mathcal{M}$.

Notemos que:

• $f^{-1}(\mathrm{\varnothing })=\mathrm{\varnothing }$ y $\mathrm{\varnothing }\in {\mathcal{B}}_n$, por tanto $\mathrm{\varnothing }\in \mathcal{M}$.
• Si $A_1,A_2,\cdots \in \mathcal{M}$, por definición $f^{-1}(A_k)\in {\mathcal{B}}_n$ para toda $k$. Pero como: $$f^{-1}(\bigcup _{k=1}^{\mathrm{\infty }}{A}_k)=\bigcup _{k=1}^{\mathrm{\infty }}{f}^{-1}(A_k).$$ Y este último pertenece a ${\mathcal{B}}_n$ (al ser unión nujmerable de elementos en ${\mathcal{B}}_n$), se tiene entonces $\bigcup _{k=1}^{\mathrm{\infty }}{A}_k\in \mathcal{M}$.
• Si $A\in \mathcal{M}$, por definición $f^{-1}(A)\in {\mathcal{B}}_n$, así que $$f^{-1}({\mathbb{R}}^m\setminus A)=E\setminus f^{-1}(A)\in {\mathcal{B}}_n.$$ Por tanto $A^c\in \mathcal{M}$.

Así que en efecto, $\mathcal{M}$ es una $\sigma$-álgebra.

Veamos ahora que $\mathcal{M}$ contiene a los conjuntos abiertos. Es aquí donde entra la hipótesis de continuidad.

Sea $U\subseteq {\mathbb{R}}^m$ un abierto. Por la continuidad de $f$, $f^{-1}(U)$ es un abierto relativo en $E$, i.e. es de la forma $E\cap H$ con $H$ abierto en ${\mathbb{R}}^n$, de modo que $f^{-1}(U)=E\cap H\in {\mathcal{B}}_n$ $⟹$ $U\in \mathcal{M}$. Lo que concluye la prueba.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Corolario. Sean $E\subseteq {\mathbb{R}}^n$ y $F\subseteq {\mathbb{R}}^m$ conjuntos de Borel. Sea $f:E\to F$ un homeomorfismo. Si $B\subseteq E$, entonces $B\in {\mathcal{B}}_n$ $⟺$ $f(B)\in {\mathcal{B}}_m$.

Más adelante…

Definiremos el concepto de función medible (aquellas funciones de las que tiene sentido «hablar de una integral»). Veremos sus principales propiedades y cómo se relacionan con los conceptos que hemos visto hasta ahora.

Tarea moral

MATERIAL EN REVISIÓN

Introducción

En las entradas pasadas vimos una gran variedad de resultados relacionados con la medida de Lebesgue y los conjuntos medibles, principalmente con la intención de aplicarlos más adelante a desarrollar una noción de integral más general. En las próximas entradas prepararemos el terreno para definir la integral de Lebesgue. Definiremos clases de conjuntos con una estructura muy particular sobre los cuales construiremos la noción de integración.

Definiciones

Notación. Como es usual, dado un conjunto $X$, denotaremos como $2^X$ a la colección de todos los subconjuntos de $X$.

Definición. Un álgebra de subconjuntos de $X$ (o sobre $X$) es una colección de conjuntos $\mathcal{M}\subseteq 2^X$ que satisface:

• $\mathrm{\varnothing }\in \mathcal{M}$
• $A,B\in \mathcal{M}$ $⟹$ $A\cup B\in \mathcal{M}$
• $A\in \mathcal{M}$ $⟹$ $A^c\in \mathcal{M}$.

Observación. Es consecuencia inmediata que:

• $X\in \mathcal{M}$ pues $X={\mathrm{\varnothing }}^c$.
• $A,B\in \mathcal{M}$ $⟹$ $A\cap B\in \mathcal{M}$ pues $A\cap B=(A^c\cup B^c{)}^c$.
• $A,B$ $⟹$ $A\setminus B\in \mathcal{M}$ pues $A\setminus B=A\cap B^c$.

Definición. Decimos que un álgebra $\mathcal{M}\subseteq 2^X$ es una $\sigma$-álgebra si además es cerrada bajo uniones numerables, es decir:

• $A_1,A_2,\cdots \in \mathcal{M}$ $⟹$ $\bigcup _{k=1}^{\mathrm{\infty }}{A}_k\in \mathcal{M}$.

Observación. Similarmente al caso anterior, se sigue de las leyes de De Morgan que $A_1,A_2,\cdots \in \mathcal{M}$ $⟹$ $\bigcap _{k=1}^{\mathrm{\infty }}{A}_k\in \mathcal{M}$.

Algunos Ejemplos de $\sigma$-álgebras

Ejemplo. Para cualquier conjunto $X$; $\mathcal{M}=2^X$ es una $\sigma$-álgebra sobre $X$.

Ejemplo. Para cualquier conjunto $X$, $\{\mathrm{\varnothing },X\}$ es una $\sigma$-álgebra (usualmente se le llama la $\sigma$-álgebra trivial).

Ejemplo. Para cualquier álgebra (o $\sigma$-álgebra) $\mathcal{M}$ sobre $X$ se tiene que $$\{\mathrm{\varnothing },X\}\subseteq \mathcal{M}\subseteq 2^X.$$

Ejemplo. Si $X={\mathbb{R}}^n$, la colección de conjuntos Lebesgue medibles, ${\mathcal{L}}_{\mathcal{n}}$, es una $\sigma$-álgebra (esto es una consecuencia inmediata de las propiedades de los conjuntos medibles). Para nuestros objetivos, éste es el ejemplo más importante.

Ejemplo (un álgebra que no es $\sigma$-álgebra). Sea $X$ un conjunto infinito. Definamos ${\mathcal{M}}_0$ como:

$$A\in {\mathcal{M}}_0⟺A\text{ es finito ó }{A}^c\text{ es finito. }$$

Veamos que ${\mathcal{M}}_0$ es un álgebra pero no una $\sigma$-álgebra. Observemos que:

• $\mathrm{\varnothing }$ es finito, de modo que $\mathrm{\varnothing }\in {\mathcal{M}}_0$.
• Notemos que si $A,B\in {\mathcal{M}}_0$ $⟹$ $A\cup B\in {\mathcal{M}}_0$ pues:
  
  • Si $A,B$ son finitos entonces $A\cup B$ es finito.
  • Si $A^c$ es finito, sin importar que ocurra con $B$, se tiene que $(A\cup B{)}^c\subseteq A^c$, de donde $(A\cup B{)}^c$ es finito. Algo similar ocurre cuando $B^c$ es finito.
• Si $A\in {\mathcal{M}}_0$, entonces alguno de $A^c$ y $(A^c{)}^c=A$ es finito, de modo que $A^c\in {\mathcal{M}}_0$.

Por todo lo anterior, se sigue que ${\mathcal{M}}_o$ es un álgebra.

Como $X$ es infinito, podemos escoger un subconjunto numerable $$S=\{x_0,x_1,x_2,x_3\dots \}.$$ Definamos $$A=\{x_0,x_2,x_4\dots \}.$$ Ni $A$ ni su complemento son finitos asi que $A\notin {\mathcal{M}}_0$. Sin embargo, $A$ se puede expresar como unión numerables de elementos en ${\mathcal{M}}_0$, a saber: $$\{x_0\},\{x_2\},\{x_4\},\dots$$

Así que ${\mathcal{M}}_0$ NO es una $\sigma$-álgebra.

$\mathrm{△}$

Ejemplo. Sea $X$ un conjunto. Definimos ${\mathcal{M}}_1\subseteq 2^X$ como:
$$A\in {\mathcal{M}}_1⟺A\text{ es numerable ó }{A}^c\text{ es numerable}.$$ Veamos que ${\mathcal{M}}_1$ es una $\sigma$-álgebra.

• $\mathrm{\varnothing }\in {\mathcal{M}}_1$ es finito, en particular numerable.
• Sean $A,B\in {\mathcal{M}}_1$. Veamos que $A\cup B\in {\mathcal{M}}_1$:
  • Si $A,B$ son numerables, entonces $A\cup B$ es numerable.
  • Si $A^c$ es numerable, entonces $(A\cup B{)}^c\subseteq A^c$, de donde $(A\cup B{)}^c$ es también numerable. Algo similar ocurre si $B^c$ es numerable.
• Si $A\in {\mathcal{M}}_1$, entonces alguno de $A^c$ y $(A^c{)}^c=A$ es numerable, de modo que $A^c\in {\mathcal{M}}_1$.

Lo anterior garantiza que ${\mathcal{M}}_1$ es un álgebra. Más aún, si $\{A_k{\}}_{k=1}^{\mathrm{\infty }}\subseteq {\mathcal{M}}_1$, entonces:

• Si $A_k$ es numerable para todo $k\in \mathbb{N}$, entonces $\bigcup _{k=1}^{\mathrm{\infty }}{A}_k$ es numerable (la unión numerable de conjuntos numerables es numerable).
• Si $A_j^c$ es numerable para al menos un $j\in \mathbb{N}$, entonces $(\bigcup _{k=1}^{\mathrm{\infty }}{A}_k{)}^c\subseteq A_j^c$, de donde $(\bigcup _{k=1}^{\mathrm{\infty }}{A}_k{)}^c$ es numerable.

En todo caso $\bigcup _{k=1}^{\mathrm{\infty }}{A}_k\in {\mathcal{M}}_1$. Concluimos que ${\mathcal{M}}_1$ es una $\sigma$-álgebra.

$\mathrm{△}$

Ejemplo. Si $X=\mathbb{R}$, definimos $\mathcal{M}$ como: $A\in \mathcal{M}$ $⟺$ $A=\mathrm{\varnothing }$ ó $A$ es una unión finita de intervalos de la forma $[a,b)$ ó $(-\mathrm{\infty },b)$ con $-\mathrm{\infty }<a<b\le \mathrm{\infty }$. Es fácil ver que $\mathcal{M}$ es un álgebra pero no una $\sigma$-álgebra (los intervalos finitos y cerrados por la derecha se pueden expresar como unión numerable de elementos en $\mathcal{M}$, pero no son elementos de $\mathcal{M}$).

Ejemplo. Cualquier álgebra finita (es decir, que solo contiene una cantidad finita de conjuntos) es en particular una $\sigma$-álgebra.

$\sigma$-álgebras a partir de otras clases de conjuntos

Proposición. La intersección (no necesariamente numerable) de $\sigma$-álgebras es una $\sigma$-álgebra.

Demostración. Sea $\{{\mathcal{M}}_i{\}}_{i\in \mathcal{I}}$ una familia de $\sigma$-álgebras sobre $X$. Veamos que $\mathcal{M}=\bigcap _{i\in \mathcal{I}}{\mathcal{M}}_i$ es una $\sigma$-álgebra.

• Como $\mathrm{\varnothing }\in {\mathcal{M}}_i$ para toda $i\in \mathcal{I}$, entonces $\mathrm{\varnothing }\in \mathcal{M}$.
• Si $A,B\in \mathcal{M}$, por definición $A,B\in {\mathcal{M}}_i$ $\mathrm{\forall }$ $i\in \mathcal{I}$ $⟹$ $A\cup B\in {\mathcal{M}}_i$ $\mathrm{\forall }i\in \mathcal{I}$ $⟹$ $A\cup B\in \mathcal{M}$.
• Si $A\in \mathcal{M}$ $⟹$ $A\in {\mathcal{M}}_i$ $\mathrm{\forall }$ $i\in \mathcal{I}$ $⟹$ $A^c\in {\mathcal{M}}_i$ $\mathrm{\forall }i\in \mathcal{I}$ $⟹$ $A^c\in \mathcal{M}$.
• Si $\{A_k{\}}_{k=1}^{\mathrm{\infty }}\subseteq \mathcal{M}$ $⟹$ $\{A_k{\}}_{k=1}^{\mathrm{\infty }}\subseteq {\mathcal{M}}_i$ $\mathrm{\forall }i\in \mathcal{I}$ $⟹$ $\bigcup _{k=1}^{\mathrm{\infty }}{A}_k\in {\mathcal{M}}_i$ $\mathrm{\forall }i\in \mathcal{I}$ $⟹$ $\bigcup _{k=1}^{\mathrm{\infty }}{A}_k\in \mathcal{M}$.

Por lo que en efecto, $\mathcal{M}$ es una $\sigma$-álgebra.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

En general, la unión de $\sigma$-álgebras no es una $\sigma$-álgebra. Veamos un ejemplo.

Ejemplo. Sea $X=\{a,b,c\}$. Podemos verificar directamente que ${\mathcal{M}}_1=\{\{a\},\{b,c\},\mathrm{\varnothing },X\}$ y ${\mathcal{M}}_1=\{\{b\},\{a,c\},\mathrm{\varnothing },X\}$ son $\sigma$-álgebras, sin embargo ${\mathcal{M}}_1\cup {\mathcal{M}}_2$ no lo es pues $\{a\},\{b\}\in {\mathcal{M}}_1\cup {\mathcal{M}}_2$ pero $\{a\}\cup \{b\}\notin {\mathcal{M}}_1\cup {\mathcal{M}}_2$.

$\mathrm{△}$

El siguiente ejemplo nos permite construir $\sigma$-álgebras a partir de colecciones arbitrarias de conjuntos. Será esencial en la siguiente entrada.

Definición. Dada $N$ una familia de subconjuntos de $X$, definimos la $\sigma$-álgebra generada por $N$, $\sigma (N)$ , como la intersección de todas las $\sigma$-álgebras que contienen a $N$.

Lo anterior tiene sentido: Por la proposición anterior, la intersección de tales $\sigma$-álgebras es una $\sigma$-álgebra. Además, existe al menos una $\sigma$-álgebra que contiene a $N$ (a saber, $2^X$). Observa que la $\sigma$-álgebra generada por $N$ contiene a $N$. Más aún, es la $\sigma$-álgebra más pequeña con tal propiedad: Cualquier otra $\sigma$-álgebra $\mathcal{M}$ con $N\subseteq \mathcal{M}$ cumple que $\sigma (N)\subseteq \mathcal{M}$ por definición.

Más adelante…

Definiremos la clase de conjuntos de Borel. Una $\sigma$-álgebra importante que será útil para definir la integral de Lebesgue más adelante.

Tarea moral

MATERIAL EN REVISIÓN

Introducción

En la entrada anterior probamos que la clase de conjuntos medibles es cerrada bajo uniones e intersecciones numerables. En esta sección usaremos dichos resultados para ver que la clase de conjuntos medibles contiene a prácticamente todos los conjuntos interesantes al menos desde un punto de vista topológico (por ejemplo abiertos, cerrados, compactos, junto con uniones e intersecciones numerables de estos). También veremos como podemos usar estos conjuntos para aproximar conjuntos medibles más generales y como es que esto caracteriza completamente a los conjuntos medibles.

Lema. Cualquier conjunto abierto $U$ se puede expresar como una unión numerable de rectángulos abiertos.

Demostración. Para cualquier $x\in U$, podemos encontrar un rectángulo abierto $Q$, tal que $x\in Q\subseteq U$. Más aún, podemos elegir $Q$ de tal manera que sus intervalos componente tengan extremos racionales (basta «encoger» un poco dichos intervalos). Si denotamos por ${\mathcal{R}}_{\mathbb{Q}}$ al conjunto de rectángulos abiertos con extremos racionales, tenemos que: $$U=\bigcup _{Q\in {\mathcal{R}}_{\mathbb{Q}};Q\subseteq U}Q.$$ La contención $\supseteq$ es obvia. La contención $\subseteq$ se sigue del hecho de que cada $p\in U$ está contenido en algún tal rectángulo. Como ${\mathcal{R}}_{\mathbb{Q}}$ es de hecho un conjunto numerable (¿Porqué?), resulta que la unión anterior es numerable. Se sigue el Lema.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Corolario. Los conjuntos abiertos, cerrados, compactos y las uniones e intersecciones numerables de estos son medibles.
Demostración. Del Lema y nuestros teoremas de cerradura, se sigue de inmediato que los abiertos son conjuntos medibles. Los cerrados (incluyendo los compactos) son medibles al ser complementos de abiertos. Las uniones e intersecciones numerables de estos son medibles, de nuevo, por nuestros teoremas de cerradura.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Los resultados anteriores nos dan una manera rápida de verificar si un conjunto es medible, aunque no nos dicen nada sobre el valor de la medida de Lebesgue. En general, suele ser complicado calcular la medida de Lebesgue de un conjunto sin usar herramientas de teoría de integración (que veremos más adelante). Veamos un ejemplo de como hacerlo directamente.

Ejercicio. Calcula la medida de Lebesgue del triángulo $$T=\{(x,y)\in {\mathbb{R}}^2:0\le x\le 1,0\le y\le x\}.$$

Solución. Claramente $T$ es cerrado así que es un conjunto medible. Fijemos $m\in \mathbb{N}$. Definamos los rectángulos: $$L_k=[\frac{k-1}{m},\frac{k}{m}]\times [0,\frac{k-1}{m}]$$ $$S_k=[\frac{k-1}{m},\frac{k}{m}]\times [0,\frac{k}{m}]$$ Para $k=1,2,\dots ,m$. Observemos que $$\bigcup _{k=1}^m{L}_k\subseteq T\subseteq \bigcup _{k=1}^m{S}_k.$$ Además los $L_k$ y los $S_k$ son ajenos salvo en conjuntos de medida cero, Luego: $$\lambda \left(\bigcup _{k=1}^m{L}_k\right)\le \lambda (T)\le \lambda \left(\bigcup _{k=1}^m{S}_k\right)$$ $$⟹\sum _{k=1}^m\lambda (L_k)\le \lambda (T)\le \sum _{k=1}^m\lambda (S_k)$$ $$⟹\sum _{k=1}^m\left(\frac{1}{m}\right)\left(\frac{k-1}{m}\right)\le \lambda (T)\le \sum _{k=1}^m\left(\frac{1}{m}\right)\left(\frac{k}{m}\right)$$ $$⟹\frac{1}{m^2}\sum _{k=1}^m(k-1)\le \lambda (T)\le \frac{1}{m^2}\sum _{k=1}^{m}k$$ $$⟹\frac{1}{m^2}\left(\frac{(m-1)(m)}{2}\right)\le \lambda (T)\le \frac{1}{m^2}\left(\frac{(m)(m+1)}{2}\right)$$ $$⟹\frac{1}{2}\frac{m-1}{m}\le \lambda (T)\le \frac{1}{2}\frac{m+1}{m}.$$ Haciendo $m⟶\mathrm{\infty }$ concluimos que $\lambda (T)=\frac{1}{2}$.

$\mathrm{△}$

Observación. El ejercicio anterior te debería de recordar un poco a las sumas de Riemann, la notación $L_k$ y $S_k$ es sugerente. Esto no es casualidad. El método anterior nos puede servir en general para probar que la medida de la región debajo de la gráfica de una función Riemann-integrable (no negativa) $G$ es igual a la intregal de Riemann. Las expresiones de las sumas inferiores y superiores nos dicen exactamente como construir colecciones de rectángulos (ajenos salvo en conjuntos de medida cero) que aproximen la región «por abajo» y «por arriba». Por monotonía dichas sumas solo se pueden acercar a $\lambda (G)$, que por definición, debe ser la integral de Riemann. Más adelante analizaremos con todo detalle esta clase de problemas.

Aproximación de conjuntos medibles

La siguiente proposición es relevante pues nos dice que podemos «aproximar» un conjunto medible con conjuntos abiertos o compactos. Estos conjuntos tienen propiedades topológicas y de convergencia muy interesantes, lo que a menudo sirve para establecer teoremas de existencia o probar estimados más fuertes.

Teorema (de aproximación de conjuntos medibles.) Si $A$ es un conjunto medible, entonces $$\lambda (A)=\underset{A\subseteq U\text{ abierto}}{inf}\{\lambda (U)\}=\underset{K\subseteq A\text{ compacto}}{sup}\{\lambda (U)\}.$$
Demostración. La primera igualdad ya la establecimos en general para la medida exterior.
Veamos la segunda igualdad: $\lambda (A)=\underset{K\subseteq A\text{ compacto}}{inf}\{\lambda (U)\}$. Consideremos primero el caso en el que $A$ es acotado. Tomemos $R$ un rectángulo cerrado suficientemente grande tal que $A$ esté contenido en el interior de $R$. Los conjuntos $A$ y $R\setminus A$ son medibles con medida finita.

Por la primera igualdad, dado $\epsilon >0$ podemos tomar un abierto $U$ tal que $R\setminus A\subseteq U$ y $$\lambda (U)<\lambda (R\setminus A)+\epsilon .$$ Notemos que $K=R\cap U^c$ es un conjunto compacto (cerrado y acotado) con $K\subseteq A$ (observa la figura), además $A\setminus K\subseteq U\setminus (R\setminus A)$. Luego, por la aditividad: $$\lambda (A)-\lambda (K)=\lambda (A\setminus K)\le \lambda (U\setminus (R\setminus A))=\lambda (U)-\lambda (R\setminus A)<\epsilon$$ $$⟹\lambda (K)\le \lambda (A)<\lambda (K)+\epsilon .$$ Como podemos hacer esto para cualquier $\epsilon >0$ concluimos que cuando $A$ es acotado se da la igualdad.

Si $A$ es de medida finita pero no necesariamente acotado, dado $\epsilon >0$, podemos tomar la sucesión: $B_k=A\cap R_k$ donde $R_k=[-k,k{]}^n$. Claramente $B_1\subseteq B_2\subseteq \dots$ y $A=\bigcup _{k=1}^{\mathrm{\infty }}{B}_k$. Por la monotonía de la medida de Lebesgue tenemos que $\lambda (B_k)\uparrow \lambda (A)$ cuando $k\to \mathrm{\infty }$, de modo que podemos encontrar algún $N$ suficientemente grande tal que $$\lambda (B_N)\le \lambda (A)<\lambda (B_N)+\frac{\epsilon }{2}.$$ Como $B_N$ es acotado, por el caso anterior, podemos encontrar algún compacto $K\subseteq B_N\subseteq A$ tal que $$\lambda (K)\le \lambda (B_N)<\lambda (K)+\frac{\epsilon }{2}.$$ Luego: $$\lambda (A)<\lambda (B_N)+\frac{\epsilon }{2}<\lambda (K)+\frac{\epsilon }{2}+\frac{\epsilon }{2}=\lambda (K)+\epsilon .$$ Como podemos hacer esto para cualquier $\epsilon >0$ concluimos que $\lambda (A)=\underset{K\subseteq A\text{ compacto}}{sup}\{\lambda (U)\}$ cuando $A$ es de medida finita.
El caso en el que $A$ es de medida infinita se queda como tarea moral.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Caracterización de conjuntos medibles

La proposición anterior es de interés pues de hecho caracteriza completamente a los conjuntos medibles con medida finita.

Lema (Completitud de la medida de Lebesgue.) Si $A\subseteq B\subseteq C$ son subconjuntos de ${\mathbb{R}}^n$, $A$ y $C$ son medibles con $\lambda (A)=\lambda (C)<\mathrm{\infty }$, entonces $B$ es medible y $\lambda (A)=\lambda (B)=\lambda (C)$.

Demostración. Nota que $C\setminus A$ es un conjunto medible con $$\lambda (C\setminus A)=\lambda (C)-\lambda (A)=0.$$ Como $B\setminus A\subseteq C\setminus A$, entonces $${\lambda }^{\ast }(B\setminus A)\le {\lambda }^{\ast }(C\setminus A)=\lambda (C\setminus A)=0.$$ $⟹B\setminus A$ es medible al ser un conjunto nulo. Como $B=A\cup (B\setminus A)$, se sigue que $B$ es medible. Por monotonía necesariamente $\lambda (A)=\lambda (B)=\lambda (C)$.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Teorema (Caracterización de conjuntos medibles). Sea $A\subseteq {\mathbb{R}}^n$ un subconjunto con ${\lambda }^{\ast }(A)<\mathrm{\infty }$. Entonces $A$ es medible si y sólo si $${\lambda }^{\ast }(A)=\underset{A\subseteq U\text{ abierto}}{inf}\{\lambda (U)\}=\underset{K\subseteq A\text{ compacto}}{sup}\{\lambda (U)\}.$$

Demostración. La implicación ($⟹$) es precisamente el teorema de aproximación. Veamos la implicación ($⟸$).

Supongamos que $\underset{A\subseteq U\text{ abierto}}{inf}\{\lambda (U)\}=\underset{K\subseteq A\text{ compacto}}{sup}\{\lambda (U)\}$, entonces, por definición de ínfimo, para cada $m=1,2,\dots$ podemos encontrar un abierto $U_m$ y un compacto $K_m$ tales que $K_m\subseteq A\subseteq U_m$ y $$\lambda (U_m)<{\lambda }^{\ast }(A)+\frac{1}{2m};$$ $${\lambda }^{\ast }(A)<\lambda (K_m)+\frac{1}{2m}.$$ Es decir $$\lambda (U_m\setminus K_m)=\lambda (U_m)-\lambda (K_m)<{\lambda }^{\ast }(A)+\frac{1}{2m}-{\lambda }^{\ast }(A)+\frac{1}{2m}=\frac{1}{m}.$$ Tomando $U_m^{\prime }=\bigcap _{j=1}^m{U}_j$ y $K_m^{\prime }=\bigcup _{j=1}^m{K}_j$, es inmediato que $K_m^{\prime }\subseteq A\subseteq U_m^{\prime }$ y $\lambda (U_m^{\prime }\setminus K_m^{\prime })\le \lambda (U_m\setminus K_m)<\frac{1}{m}$, así que podemos suponer sin pérdida de generalidad que $U_1\supseteq U_2\supseteq \dots$ y $K_1\subseteq K_2\subseteq \dots$.

Definamos $F=\bigcap _{j=1}^{\mathrm{\infty }}{U}_j$ y $G=\bigcup _{j=1}^{\mathrm{\infty }}{K}_j$. Estos son medibles al ser uniones e intersecciones numerables de conjuntos medibles respectivamente. Claramente $G\subseteq A\subseteq F$, además $$F\setminus G=(\bigcap _{j=1}^{\mathrm{\infty }}{U}_j)\cap (\bigcup _{j=1}^{\mathrm{\infty }}{K}_j{)}^c=(\bigcap _{j=1}^{\mathrm{\infty }}{U}_j)\cap (\bigcap _{j=1}^{\mathrm{\infty }}{K}_j^c)=\bigcap _{j=1}^{\mathrm{\infty }}(U_j\cap K_j^c)$$ $$=\bigcap _{j=1}^{\mathrm{\infty }}(U_j\setminus K_j).$$ Notemos que $U_1\setminus K_1\supseteq U_2\setminus K_2\supseteq \dots$ y $U_1\setminus K_1$ es de medida finita. Se sigue por monotonía de la medida de Lebesgue: $$\lambda (U_j\setminus K_j)\downarrow \lambda (F\setminus G).$$ Pero como $0\le \lambda (U_j\setminus K_j)<\frac{1}{j}$ para $j=1,2,\dots$ la única posibilidad es que $\lambda (U_j\setminus K_j)\to 0$ cuando $j\to \mathrm{\infty }$. Luego $$\lambda (F\setminus G)=0.$$ $$⟹\lambda (F)=\lambda (G)+\lambda (F\setminus G)=\lambda (G).$$
Como $G\subseteq A\subseteq F$; $G$ y $F$ son medibles con medida finita y $\lambda (F)=\lambda (G)$, se sigue por el Lema anterior que $A$ es medible.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Siguiendo la línea de la proposición anterior, veamos un par de equivalencias más para conjuntos medibles.

Teorema (Equivalencia de conjuntos medibles). Las siguientes son equivalentes.

1. $A$ es un conjunto medible.
2. Para cualquier $\epsilon >0$ existe un abierto $U$ tal que $A\subseteq U$ y ${\lambda }^{\ast }(U\setminus A)<\epsilon$.
3. Para cualquier $\epsilon >0$ existe un cerrado $K$ tal que $K\subseteq A$ y ${\lambda }^{\ast }(A\setminus K)<\epsilon$

Demostración. Probaremos la equivalencia ($1⟺2$). La tercera se queda como tarea moral.

Si $A$ es de medida finita, la implicación (1$⟹$2) es consecuencia inmediata del teorema anterior.

Si $A$ es de medida infinita, podemos partirlo en una cantidad numerable de conjuntos medibles ajenos con medida finita: $A=\bigcup _{k=1}^{\mathrm{\infty }}{B}_k$ (piensa por ejemplo en intersecar $A$ con cada rectángulo semiabierto de la forma $[p_1,p_1+1)\times [p_2,p_2+1)\times \cdots \times [p_n,p_n+1)$ donde $p_1,p_2,\dots p_n$ son todos enteros).

Para cada $B_k$, podemos encontrar un abierto $U_k$ tal que $B_k\subseteq U_k$ y $\lambda (U_k\setminus B_k)<\frac{\epsilon }{2^k}$. Sea $U=\bigcup _{k=1}^{\mathrm{\infty }}{U}_k$. Éste es abierto con $A\subseteq U$, además tenemos $U\setminus A\subseteq \bigcup _{k=1}^{\mathrm{\infty }}(U_k\setminus B_k)$. Por monotonía y subaditividad: $$\lambda (U\setminus A)\le \sum _{k=1}^{\mathrm{\infty }}\lambda (U_k\setminus B_k)<\sum _{k=1}^{\mathrm{\infty }}\frac{\epsilon }{2^k}=\epsilon .$$
Con esto concluimos que (1$⟹$2).
Ahora supongamos 2. En particular para cada $k=1,2,\dots$ podemos encontrar un abierto $U_k$ tal que $A\subseteq U_k$ y ${\lambda }^{\ast }(U_k\setminus A)<\frac{1}{k}$. Más aún, imitando el argumento de la equivalencia anterior, podemos suponer sin pérdida de generalidad que $U_1\supseteq U_2\supseteq \dots$. Consideremos $G=\bigcap _{k=1}^{\mathrm{\infty }}{U}_k$. Éste es medible con $A\subseteq G$, además $G\setminus A\subseteq U_k\setminus A$ para todo $k=1,2,\dots$ por lo que $${\lambda }^{\ast }(G\setminus A)\le {\lambda }^{\ast }(U_k\setminus A)<\frac{1}{k}\mathrm{\forall }k=1,2,\dots$$ De donde $${\lambda }^{\ast }(G\setminus A)=0.$$ Así que $G$ y $G\setminus A$ son medibles (éste último al ser nulo). Se sigue que $A=G\setminus (G\setminus A)$ es medible como queríamos probar.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Más adelante…

Estudiaremos la estructura «conjuntista» de los conjuntos medibles. Esto será de utilidad, por un lado para definir rápidamente el concepto de función medible; pero también para construir nociones de integración sobre espacios abstractos como veremos al final del curso.

Tarea moral

• Completa la demostración del teorema de aproximación de conjuntos medibles. [
  Sugerencia: Imita el argumento en el caso en el que $A$ es de medida finita, encontrando compactos con medida arbitrariamente grande contenidos en $A$].
• Demuestra la equivalencia del punto 3. en el Teorema de equivalencia de conjuntos medibles. [Sugerencia: Puedes usar la equivalencia ($1⟺2$) con el complemento de $A$].

MATERIAL EN REVISIÓN

Introducción.

En la entrada pasada vimos dos ejemplos importantes de conjuntos medibles y algunas resultados básicos de operaciones con conjuntos. En esta entrada veremos algunos resultados similares, pero ahora con una cantidad numerable de elementos. Estableceremos también la aditividad numerable de la medida de Lebesgue y un par de resultados relacionados.

Proposición. Si $A_1,A_2,\dots$ es una familia numerable de conjuntos medibles ajenos dos a dos, entonces $A=\bigcup _{k=1}^{\mathrm{\infty }}{A}_k$ es medible.

Demostración. Definamos $U_0=\mathrm{\varnothing }$ y para cada $m=1,2,\dots$, $U_m=\bigcup _{k=1}^m{A}_k$. Por la cerradura de la clase de conjuntos medibles bajo uniones finitas, sabemos que $U_m$ es medible para todo $m\in \mathbb{N}$. Como $A_m$ es medible, para cualquier $E\subseteq {\mathbb{R}}^n$:
$$\begin{array}{rl}{\lambda }^{\ast }(E\cap U_m)& ={\lambda }^{\ast }(E\cap U_m\cap A_m)+{\lambda }^{\ast }(E\cap U_m\cap A_m^c)\\ & ={\lambda }^{\ast }(E\cap A_m)+\lambda (E\cap U_{m-1}).\end{array}$$ Luego, por inducción se sigue que: $${\lambda }^{\ast }(E\cap U_m)=\sum _{k=1}^m{\lambda }^{\ast }(E\cap A_k).$$ Como $U_m$ es medible: $$\begin{array}{rl}{\lambda }^{\ast }(E)& ={\lambda }^{\ast }(E\cap U_m)+{\lambda }^{\ast }(E\cap U_m^c)\\ & \ge \sum _{k=1}^m{\lambda }^{\ast }(E\cap A_k)+{\lambda }^{\ast }(E\cap A^c).\end{array}$$ La última desigualdad se cumple pues $U_m\subseteq A$. Haciendo tender $m\to \mathrm{\infty }$ en la expresión anterior tenemos:
$$\begin{array}{rl}{\lambda }^{\ast }(E)& \ge \sum _{k=1}^{\mathrm{\infty }}{\lambda }^{\ast }(E\cap A_k)+{\lambda }^{\ast }(E\cap A^c)\\ & \ge {\lambda }^{\ast }(\bigcup _{k=1}^{\mathrm{\infty }}(E\cap A_k))+{\lambda }^{\ast }(E\cap A^c)\\ & ={\lambda }^{\ast }(E\cap A)+{\lambda }^{\ast }(E\cap A^c)\\ & \ge {\lambda }^{\ast }(E).\end{array}$$ (La segunda y última desigualdad se tienen por subaditividad). Luego, las desigualdades anteriores son de hecho igualdades. En particular $${\lambda }^{\ast }(E)={\lambda }^{\ast }(E\cap A)+{\lambda }^{\ast }(E\cap A^c).$$ Como esto es cierto para cualquier $E\subseteq {\mathbb{R}}^n$, se sigue que $A$ es medible.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Observación. Al tomar $E=A$ en la última cadena de (des)igualdades en la proposición anterior podemos deducir:
$$\lambda (A)={\lambda }^{\ast }(A)=\sum _{k=1}^{\mathrm{\infty }}{\lambda }^{\ast }(A_k)=\sum _{k=1}^{\mathrm{\infty }}\lambda (A_k).$$
Esto es precisamente la aditividad numerable. Lo enunciaremos como una proposición.

Proposición (Aditividad numerable o Sigma-aditividad). Si $A_1,A_2,\dots$ es una familia numerable de conjuntos medibles ajenos dos a dos, entonces:
$$\lambda (A)=\sum _{k=1}^{\mathrm{\infty }}\lambda (A_k).$$

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Proposición. Si $A_1,A_2\dots$ es una familia de conjuntos medibles (no necesariamente ajenos) entonces $A=\bigcup _{k=1}^{\mathrm{\infty }}{A}_k$ es medible.

Demostración. La idea es reducirlo al caso de conjuntos ajenos con una elección adecuada de conjuntos. A ésta técnica se le conoce comúnmente como ajenización.

Definamos $B_1=A_1$ y $B_m=A_m\setminus (\bigcup _{k=1}^{m-1}{A}_k)$ para $m\ge 2$. Cada $B_m$ es medible (por propiedades de los conjuntos medibles). Además, es fácil verificar usando inducción que $B_1,B_2\dots$ es una familia de conjuntos ajenos dos a dos y que para cada $m=1,2,\dots$, $$\bigcup _{k=1}^m{A}_k=\bigcup _{k=1}^m{B}_k.$$ Así pues $A=\bigcup _{k=1}^{\mathrm{\infty }}{A}_k=\bigcup _{k=1}^{\mathrm{\infty }}{B}_k$ es medible por la proposición anterior.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Usando el resultado anterior y las leyes de De Morgan se sigue fácilmente:

Proposición. Si $A_1,A_2\dots$ es una familia de conjuntos medibles, entonces $A=\bigcap _{k=1}^{\mathrm{\infty }}{A}_k$ es medible.

La demostración se queda como tarea moral.

Proposición (Monotonía de la medida de Lebesgue).

1. Si $A_k$ es una sucesión creciente de conjuntos medibles, i.e. $A_1\subseteq A_2\subseteq \dots$, entonces $$\lambda \left(\bigcup _{k=1}^{\mathrm{\infty }}{A}_k\right)=\underset{k\to \mathrm{\infty }}{lim}\lambda (A_k).$$
2. Si $A_k$ es una sucesión decreciente de conjuntos medibles, i.e. $A_1\supseteq A_2\supseteq \dots$ y $\lambda (A_1)\le \mathrm{\infty }$, entonces $$\lambda \left(\bigcap _{k=1}^{\mathrm{\infty }}{A}_k\right)=\underset{k\to \mathrm{\infty }}{lim}\lambda (A_k).$$

Demostración. 1. Por la cerradura de conjuntos medibles bajo uniones numerables, claramente el conjunto en cuestión es medible así que tiene sentido hablar de su medida de Lebesgue. Si $\lambda (A_N)=\mathrm{\infty }$ para algún $N$, entonces la igualdad se da de manera obvia pues necesariamente $\lambda (A_k)=\mathrm{\infty }$ para $k\ge N$. Así que podemos suponer sin pérdida de generalidad que $\lambda (A_k)<\mathrm{\infty }$ para todo $k$.
Nuevamente podemos ajenizar: Definamos $U_1=A_1$, $U_m=A_m\setminus (\bigcup _{k=1}^{m-1}{A}_k)=A_m\setminus A_{m-1}$ (en la última igualdad usamos que la sucesión es creciente). De nuevo se satisface que $U_1,U_2\dots$ es una colección de conjuntos medibles ajenos con $\bigcup _{k=1}^m{U}_k=\bigcup _{k=1}^m{A}_k$. Se sigue entonces por la aditividad numerable: $$\lambda \left(\bigcup _{k=1}^{\mathrm{\infty }}{A}_k\right)=\lambda \left(\bigcup _{k=1}^{\mathrm{\infty }}{U}_k\right)=\sum _{k=1}^{\mathrm{\infty }}\lambda (U_k)=\underset{m\to \mathrm{\infty }}{lim}\sum _{k=1}^m\lambda (U_k).$$
Ahora, como $U_m=A_m\setminus A_{m-1}$ para $m\ge 2$ y los $A_m$ son de medida finita, tenemos que $\lambda (U_m)=\lambda (A_m)-\lambda (A_{m-1})$, de modo que la suma $\sum _{k=1}^m\lambda (U_k)$ es simplemente $\lambda (A_m)$. Sustituyendo en la expresión anterior:
$$\lambda (\bigcup _{k=1}^{\mathrm{\infty }}{A}_k)=\underset{m\to \mathrm{\infty }}{lim}\sum _{k=1}^m\lambda (U_k)=\underset{m\to \mathrm{\infty }}{lim}\lambda (A_m).$$ Como queríamos probar.

2. Similarmente al caso anterior, el conjunto $\bigcap _{k=1}^{\mathrm{\infty }}{A}_k$ es medible. Definamos $V_m=A_1\setminus A_m$, de modo que $V_1,V_2,\dots$ es una sucesión creciente de conjuntos medibles, donde cada conjunto está contenido en $A_1$. Por el inciso anterior se sigue que: $$\lambda \left(\bigcup _{k=1}^{\mathrm{\infty }}{V}_k\right)=\underset{k\to \mathrm{\infty }}{lim}\lambda (V_k)=\underset{k\to \mathrm{\infty }}{lim}[\lambda (A_1)-\lambda (A_k)]=\lambda (A_1)-\underset{k\to \mathrm{\infty }}{lim}\lambda (A_k).$$
De las definiciones se sigue fácilmente que $\bigcup _{k=1}^{\mathrm{\infty }}{V}_k=A_1\setminus (\bigcap _{k=1}^{\mathrm{\infty }}{A}_k)$, de donde $$\lambda \left(\bigcup _{k=1}^{\mathrm{\infty }}{V}_k\right)=\lambda (A_1)-\lambda \left(\bigcap _{k=1}^{\mathrm{\infty }}{A}_k\right).$$ Combinando las dos igualdades anteriores concluimos $$\lambda \left(\bigcap _{k=1}^{\mathrm{\infty }}{A}_k\right)=\underset{n\to \mathrm{\infty }}{lim}\lambda (A_k).$$

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Observación. El límite en el inciso 1 es un límite creciente, que denotaremos como $\lambda (A_k)\uparrow \lambda (\bigcup _{k=1}^{\mathrm{\infty }}{A}_k)$, mientras que en el inciso 2 es un límite decreciente que denotaremos como $\lambda (A_k)\downarrow \lambda (\bigcap _{k=1}^{\mathrm{\infty }}{A}_k)$. En general la hipótesis de que $A_1$ (o al menos algún $A_m$) sea de medida finita no se puede relajar. Considera por ejemplo la sucesión decreciente de conjuntos $A_m=[m,\mathrm{\infty })$. Cada uno de estos es medible al ser una unión numerable de intervalos cerrados, sin embargo $\lambda (A_m)=\mathrm{\infty }$ para todo $m$ mientras que $\bigcap _{k=1}^{\mathrm{\infty }}{A}_k=\mathrm{\varnothing }$, así que en este caso: $$\underset{m\to \mathrm{\infty }}{lim}\lambda (A_m)=\mathrm{\infty }\ne 0=\lambda (\mathrm{\varnothing })=\lambda (\bigcap _{k=1}^{\mathrm{\infty }}{A}_k).$$

Más adelante…

Continuaremos con nuestro estudio de los conjuntos medibles. Veremos algunas definiciones alternas de conjunto medible y cómo es que podemos «aproximar» estos conjuntos por otros más sencillos.

Tarea moral

• Demuestra que Si $A_1,A_2\dots$ es una familia de conjuntos medibles, entonces $A=\bigcap _{k=1}^{\mathrm{\infty }}{A}_k$ es medible.

MATERIAL EN REVISIÓN

Introducción

En las secciones pasadas comentamos que existen conjuntos con comportamientos muy «patológicos» respecto a la medida exterior. Vimos ejemplos donde la aditivad no se satisface, una propiedad deseable en cualquier noción de medida. Una posible manera de arreglar este problema es, en vez de buscar definir la medida sobre todos los subconjuntos de ${\mathbb{R}}^n$, restringirnos únicamente a los «conjuntos bien portados» respecto a la medida exterior (esperando que esta clase de conjuntos sea lo suficientemente general). Ésta es precisamente la idea de conjunto medible.

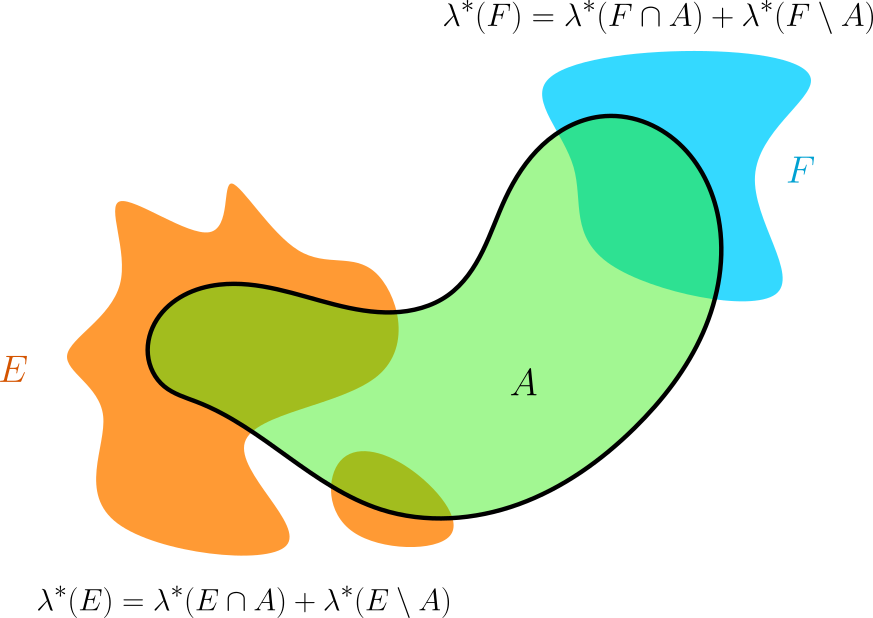
Definición. Sea $A$ un subconjunto de ${\mathbb{R}}^n$. Decimos que $A$ es Lebesgue medible o simplemente medible si y sólo si para cualquier $E\subseteq {\mathbb{R}}^n$,
$${\lambda }^{\ast }(E)={\lambda }^{\ast }(E\cap A)+{\lambda }^{\ast }(E\setminus A)$$ Si $A$ es medible, definimos su medida de Lebesgue, $\lambda (A)$, como su medida exterior: $$\lambda (A)={\lambda }^{\ast }(A).$$

Es conveniente pensar a los conjuntos medibles como aquellos «bien portados» bajo la aditividad.

Existen varias definiciones equivalentes de conjuntos medibles. Más adelante veremos algunas de ellas.

Observación. Recordando que $E\setminus A=E\cap A^c$, la igualdad en la definición de conjunto medible se puede escribir de forma alternativa como $${\lambda }^{\ast }(E)={\lambda }^{\ast }(E\cap A)+{\lambda }^{\ast }(E\cap A^c).$$ Usaremos una u otra según convenga.

Observación. Para probar que un conjunto $A$ es medible, hay que probar que ${\lambda }^{\ast }(E)={\lambda }^{\ast }(E\cap A)+{\lambda }^{\ast }(E\setminus A)$ para cualquier $E\subseteq {\mathbb{R}}^n$. Observa que, por subaditividad, siempre es cierto que ${\lambda }^{\ast }(E)\le {\lambda }^{\ast }(E\cap A)+{\lambda }^{\ast }(E\setminus A)$. Así que es suficiente probar la desigualdad opuesta. Más aún, si $E$ es de medida exterior infinita, dicha desigualdad se da de manera trivial, por tanto, basta suponer que $E$ es de medida exterior finita. En resúmen $A$ es medible si y sólo si para cualquier $E$ con ${\lambda }^{\ast }(E)<\mathrm{\infty }$, se satisface $${\lambda }^{\ast }(E\cap A)+{\lambda }^{\ast }(E\setminus A)\le {\lambda }^{\ast }(E).$$

No es inmediato ver que siquiera existen conjuntos medibles. Veremos primero dos ejemplos sencillos pero muy importantes. Más adelante probaremos que la clase de conjuntos medibles es cerrada bajo una gran cantidad de operaciones con conjuntos (complementos, uniones e intersecciones finitas y numerables, entre otros) lo que garantizará que la clase de conjuntos medibles es de hecho bastante general.

Dos ejemplos importantes de conjuntos medibles.

Definición. Decimos que un conjunto $N\subseteq {\mathbb{R}}^n$ es nulo si tiene medida exterior cero.

Proposición. Los conjuntos nulos son medibles.

Demostración. Sea $N\subseteq {\mathbb{R}}^n$ un conjunto con ${\lambda }^{\ast }(N)=0$ y $E\subseteq {\mathbb{R}}^n$ un conjunto arbitrario. Por subaditividad ya sabemos que ${\lambda }^{\ast }(E)\le {\lambda }^{\ast }(E\cap N)+{\lambda }^{\ast }(E\setminus N)$ así que basta probar la desigualdad opuesta.

Por monotonía tenemos $0\le {\lambda }^{\ast }(N\cap E)=\le {\lambda }^{\ast }(N)=0$ y ${\lambda }^{\ast }(E\setminus N)\le {\lambda }^{\ast }(E)$ , luego:
$${\lambda }^{\ast }(E\cap N)+{\lambda }^{\ast }(E\setminus N)=0+{\lambda }^{\ast }(E\setminus N)\le {\lambda }^{\ast }(E)$$ Como lo anterior es cierto para cualquier $E\subseteq {\mathbb{R}}^n$, concluimos que $N$ es medible.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Proposición. Los semiespacios, es decir, conjuntos de la forma $$H_i^{\pm }=\{(x_1,x_2,\dots ,x_n)\in {\mathbb{R}}^n:\pm x_i\ge 0\}$$ Son medibles.

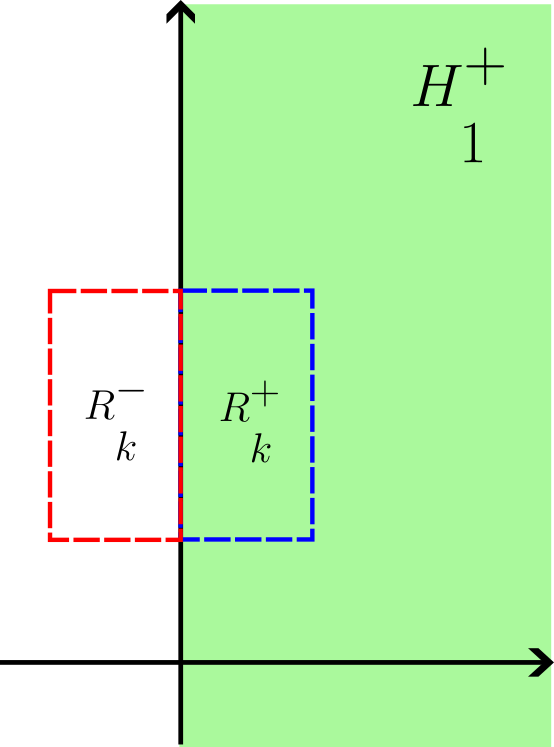
Demostración. Probaremos que $H_1^{+}$ es medible. Los demás casos son idénticos. Como ya observamos, es suficiente probar que ${\lambda }^{\ast }(E\cap A)+{\lambda }^{\ast }(E\setminus A)\le {\lambda }^{\ast }(E)$ para cualquier $E$ con ${\lambda }^{\ast }(E)<\mathrm{\infty }$.

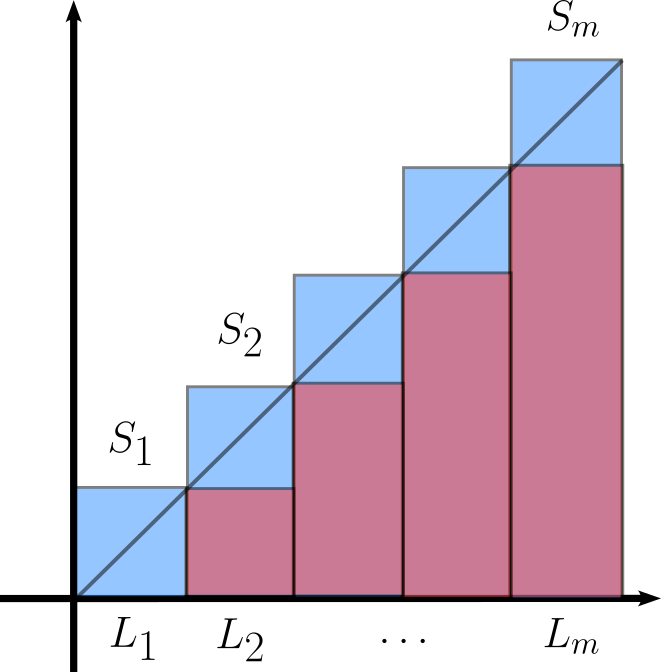
Sea entonces $E\subseteq {\mathbb{R}}^n$ con ${\lambda }^{\ast }(E)<\mathrm{\infty }$ arbitrario. Dado $\epsilon >0$ arbitrario, tomemos una cubierta con rectángulos cerrados $E\subseteq \bigcup _{k=1}^{\mathrm{\infty }}{R}_k$ tal que $${\lambda }^{\ast }(E)\le \sum _{k=1}^{\mathrm{\infty }}|R_k|<{\lambda }^{\ast }(E)+\epsilon .$$ Si hay algún rectángulo «partido» por el semiespacio, es decir, $R_k=[a_1^k,b_1^k]\times [a_2^k,b_2^k]\times \cdots \times [a_n^k,b_n^k].$ Con $a_1^k<0<b_1^k$, podemos reemplazarlo por los dos subrectángulos $R_k^{-}=[a_1^k,0]\times [a_2^k,b_2^k]\times \cdots \times [a_n^k,b_n^k]$ y $R_k^{+}=[0,b_1^k]\times [a_2^k,b_2^k]\times \cdots \times [a_n^k,b_n^k]$ como en la figura (nota que $|R_k|=|R_k^{+}|+|R_k^{-}|$). Así que podemos suponer sin pérdidad de generalidad que para cualquier $k=1,2,\dots$, o bien $R_k\subseteq H_1^{+}$ o bien $R_k\subseteq H_1^{-}$.

Si denotamos por $I^{+}$ al conjunto de índices $k$ tales que $R_k\subseteq H_1^{+}$ y por $I^{-}$ al conjunto de índices $k$ tales que $R_k\cap (H_1^{+}{)}^c\ne \mathrm{\varnothing }$, claramente:
$$E\cap H_1^{+}\subseteq \bigcup _{k\in I^{+}}{R}_k;E\setminus H_1^{+}\subseteq \bigcup _{k\in I^{-}}{R}_k$$ De donde:
$$\begin{array}{rl}{\lambda }^{\ast }(E\cap H_1^{+})+{\lambda }^{\ast }(E\setminus H_1^{+})& \le \sum _{k\in I^{+}}|R_k|+\sum _{j\in I^{-}}|R_j|\\ & \le \sum _{k=1}^{\mathrm{\infty }}|R_k|\\ & <{\lambda }^{\ast }(E)+\epsilon \end{array}$$

Como esto es cierto para cualquier $\epsilon >0$, necesariamente $${\lambda }^{\ast }(E\cap H_1^{+})+{\lambda }^{\ast }(E\setminus H_1^{+})\le {\lambda }^{\ast }(E).$$

Concluimos que $H_1^{+}$ es un conjunto medible.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Conjuntos medibles y operaciones con conjuntos

Veamos que la clase de conjuntos medibles es cerrada bajo operaciones «básicas» con conjuntos. Más adelante veremos algunos resultados más fuertes.

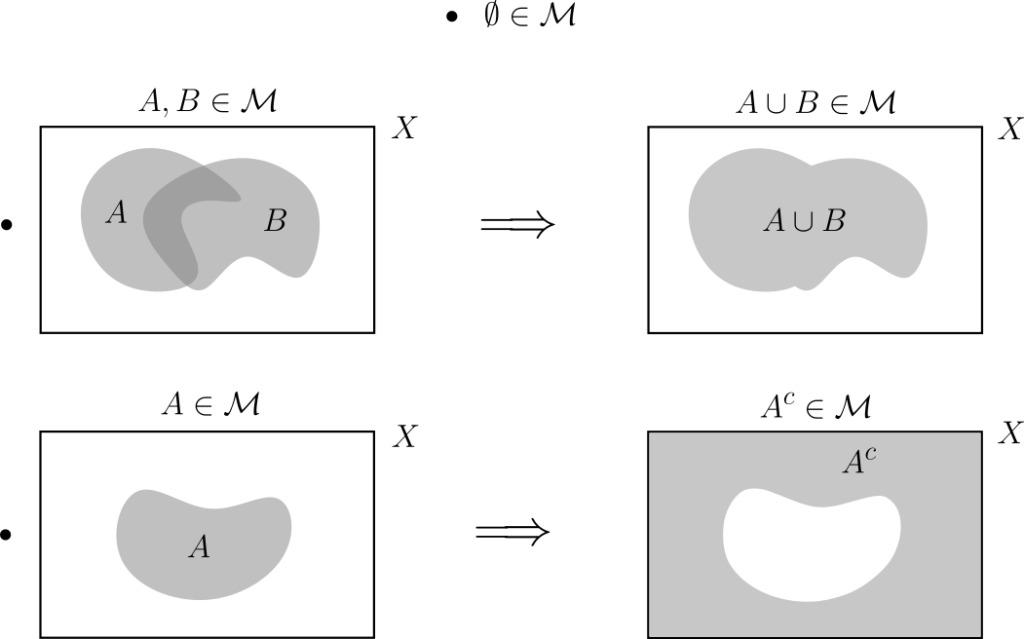
Proposición (Propiedades de conjuntos medibles).

1. Si $A$ es medible, entonces su complemento $A^c={\mathbb{R}}^n\setminus A$ es medible.
2. Si $A$ es medible y $x\in {\mathbb{R}}^n$, entonces la traslación $x+A$ es medible.
3. Si $A_1,A_2,\dots A_m$ son medibles, entonces $\bigcup _{k=1}^m{A}_k$ y $\bigcap _{k=1}^m{A}_k$ son medibles.

Demostración. 1. es inmediato gracias a la simetría de la definición.

Por un argumento de doble contención es fácil verificar que para cualquier $E\subseteq {\mathbb{R}}^n$, $$E\cap (x+A)=x+((-x+E)\cap A)$$ Y $$E\setminus (x+A)=x+((-x+E)\setminus A).$$ Usando la invarianza bajo traslaciones de la medida exterior y el hecho de que $A$ es medible:

$$\begin{array}{rl}{\lambda }^{\ast }(E)& ={\lambda }^{\ast }(-x+E)\\ & ={\lambda }^{\ast }((-x+E)\cap A)+{\lambda }^{\ast }((-x+E)\setminus A)\\ & ={\lambda }^{\ast }(x+(-x+E)\cap A)+{\lambda }^{\ast }(x+(-x+E)\setminus A)\\ & ={\lambda }^{\ast }(E\cap (x+A))+{\lambda }^{\ast }(E\setminus (x+A)).\end{array}$$ Se sigue 2.

Probaremos 3. para el caso en el que tenemos solamente dos conjuntos. El caso general se sigue fácilmente por inducción. Sean entonces $A$ y $B$ conjuntos medibles y $E\subseteq {\mathbb{R}}^n$ cualquier conjunto con medida exterior finita. Usando la definición sobre $A$, $B$, y las leyes de De Morgan:

$$\begin{array}{rl}{\lambda }^{\ast }(E)& ={\lambda }^{\ast }(E\cap A)+{\lambda }^{\ast }(E\cap A^c)\\ & ={\lambda }^{\ast }((E\cap A)\cap B)+{\lambda }^{\ast }((E\cap A)\cap B^c)+{\lambda }^{\ast }((E\cap A^c)\cap B)+{\lambda }^{\ast }((E\cap A^c)\cap B^c)\\ & ={\lambda }^{\ast }(E\cap A\cap B)+{\lambda }^{\ast }(E\cap A\cap B^c)+{\lambda }^{\ast }(E\cap A^c\cap B)+{\lambda }^{\ast }(E\cap (A\cup B{)}^c).\end{array}$$

Por otro lado, como $A\cup B=(A\cap B)\cup (A\cap B^c)\cup (A^c\cap B)$, tenemos por subaditividad:
$${\lambda }^{\ast }(E\cap (A\cup B))\le {\lambda }^{\ast }(E\cap (A\cap B))+{\lambda }^{\ast }(E\cap (A\cap B^c))+{\lambda }^{\ast }(E\cap (A^c\cap B)).$$
Finalmente, usando las desigualdades anteriores tenemos:

$$\begin{array}{rl}& {\lambda }^{\ast }(E\cap (A\cup B))+{\lambda }^{\ast }(E\cap (A\cup B{)}^c)\\ & \le {\lambda }^{\ast }(E\cap (A\cap B))+{\lambda }^{\ast }(E\cap (A\cap B^c))+{\lambda }^{\ast }(E\cap (A^c\cap B))+{\lambda }^{\ast }(E\cap A^c\cap B^c)\\ & ={\lambda }^{\ast }(E).\end{array}$$
Concluimos (por la observación) que $A\cup B$ es un conjunto medible. Para el caso de la intersección simplemente observamos que: $A\cap B=(A^c\cup B^c{)}^c$. Éste último siendo medible por las propiedades anteriores.

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

La proposición anterior nos asegura que la clase de conjuntos medibles es cerrada bajo operaciones básicas con conjuntos, más adelante veremos versiones más fuertes de estos resultados. Ahora estamos en condiciones de dar un par de ejemplos esperables de conjuntos medibles, además de algunos resultados relacionados con la aditividad.

Corolario. Los rectángulos cerrados y abiertos son conjuntos medibles.
Demostración. Los rectángulos cerrados se pueden ver como una intersección finita de semiespacios trasladados. Los rectángulos abiertos se pueden ver como una intersección finita de complementos de semiespacios cerrados (es decir «semiespacios» abiertos).

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Veamos ahora que la medida de Lebesgue sobre los conjuntos medibles es aditiva. Una de las grandes ventajas de este concepto.

Proposición (Aditividad de la Medida de Lebesgue).

1. Si $A_1,A_2,\dots ,A_n$ son conjuntos medibles ajenos dos a dos, entonces $$\lambda (\bigcup _{k=1}^n{A}_k)=\sum _{k=1}^n\lambda (A_k).$$
2. Si $B\subseteq A$ y $B$ es de medida finita entonces $$\lambda (A\setminus B)=\lambda (A)-\lambda (B).$$

Demostración. Por las proposiciones anteriores, todos los conjuntos en cuestión son medibles, así que tiene sentido hablar de su medida de Lebesgue.

Probaremos 1. solamente para dos conjuntos ajenos. El caso general se sigue por inducción. Para ello simplemente notemos que:
$$\begin{array}{rl}\lambda (A\cup B)& ={\lambda }^{\ast }(A\cup B)\\ & ={\lambda }^{\ast }((A\cup B)\cap A)+{\lambda }^{\ast }((A\cup B)\cap A^c)\\ & ={\lambda }^{\ast }(A)+{\lambda }^{\ast }(B)\\ & =\lambda (A)+\lambda (B).\end{array}$$
En la segunda igualdad se usó la definición de que $A$ es medible.

Para 2. notemos que $A$ es la unión ajena de $B$ y $A\setminus B$. Se sigue por el inciso anterior que
$$\lambda (A)=\lambda (B)+\lambda (A\setminus B)$$ $$⟹\lambda (A\setminus B)=\lambda (A)-\lambda (B).$$
(La restricción de que $B$ sea de medida finita es simplemente para que la expresión $\lambda (A)-\lambda (B)$ tenga sentido).

$\mathrm{<img\; draggable="false"\; role="img"\; class="emoji"\; alt="◻"\; src="https://s.w.org/images/core/emoji/14.0.0/svg/25fb.svg">}$

Más adelante…

Seguiremos con nuestro estudio de los conjuntos medibles. Veremos que los conjuntos medibles son cerrados bajo otras operaciones con conjuntos (por ejemplo uniones numerables), lo que nos permitirá probar que la clase de conjuntos medibles es de hecho bastante general.

Tarea moral

• Prueba que si $A,B$ son conjunto medibles, entonces $A\setminus B$ es un conjunto medible.