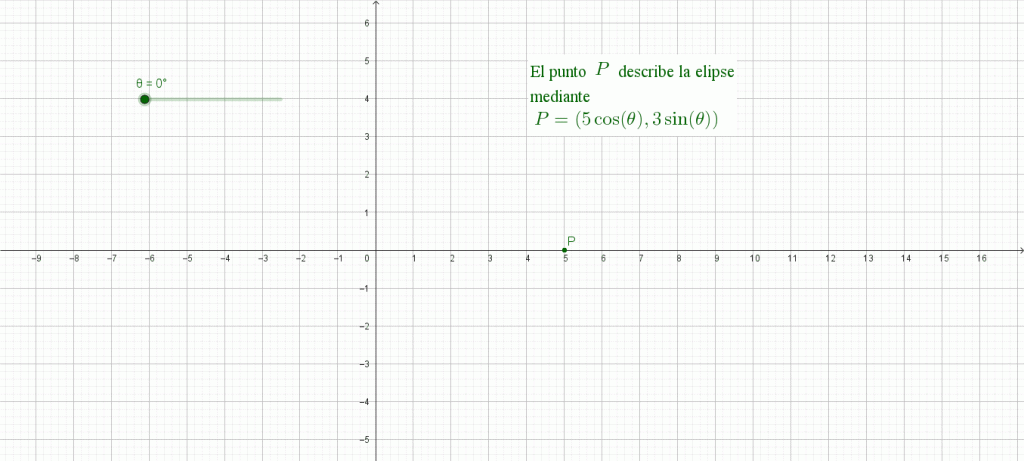
Elipse

La elipse : $${\displaystyle \frac{x^2}{a^2}}+{\displaystyle \frac{y^2}{b^2}}=1$$

se puede parametrizar como

$$\{\genfrac{}{}{0ex}{}{x=a\cos \theta }{y=b\sin \theta }$$

ya que si elevamos al cuadrado ambas ecuaciones obtenemos que $$\{\genfrac{}{}{0ex}{}{x^2=a^2{\cos }^2\theta }{y^2=b^2{\sin }^2\theta }$$ luego, despejando y sumando miembro a miembro observamos que $${\displaystyle \frac{x^2}{a^2}}+{\displaystyle \frac{y^2}{b^2}}={\cos }^2\theta +{\sin }^2\theta =1$$ que es la ecuación de la elipse.

En esta imagen puedes observar una animación de la parametrización.

Hipérbola

La hipérbola: $$x^2–y^2=1$$

se puede parametrizar como

$$\{\genfrac{}{}{0ex}{}{x=\sec \theta }{y=\tan \theta }$$

ya que si elevamos al cuadrado cada ecuación tenemos que $$\{\genfrac{}{}{0ex}{}{x^2={\sec }^2\theta }{y^2={\tan }^2\theta }$$ luego, restándolas vemos que $$x^2–y^2={\sec }^2\theta –{\tan }^2\theta ={\displaystyle \frac{1}{{\cos }^2\theta }}–{\displaystyle \frac{{\sin }^2\theta }{{\cos }^2\theta }}={\displaystyle \frac{1–{\sin }^2\theta }{{\cos }^2\theta }}={\displaystyle \frac{{\cos }^2\theta }{{\cos }^2\theta }}=1$$ obtenemos la ecuación de la hipérbola.

Otra manera de parametrizar la hipérbola es considerando

$$\{\genfrac{}{}{0ex}{}{x=\cosh \theta ={\displaystyle \frac{e^t+e^{-t}}{2}}}{y=\sinh \theta ={\displaystyle \frac{e^t–e^{-t}}{2}}}$$

$$x^2–y^2={\cosh }^2\theta –{\sinh }^2\theta =1$$

En la siguiente imagen puedes observar una animación de la hipérbola.

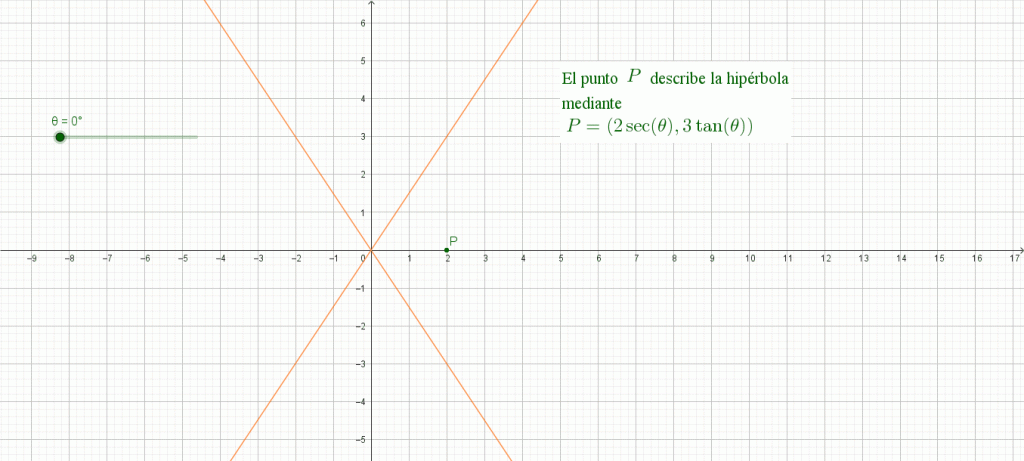
Longitud de arco

Consideramos una curva parametrizada $$\alpha :[a,b]\subset \mathbb{R}\to {\mathbb{R}}^2$$ $$\alpha (t)=(x(t),y(t))$$

Sean $P=\alpha (a)$

y $Q=\alpha (b)$

¿Cuál es la longitud de arco desde $P$ hasta $Q$?

• Aproximemos la longitud de la curva como suma de segmentos de recta.

$\sum _{i=1}^n\Vert \alpha (t_i)–\alpha (t_{i-1})\Vert$ con la partición $a=t_0<t_1<\cdots <t_n=b$

Nos preguntamos si hay un teorema del valor medio. Es decir, existe $\rho \in (a,b)$ tal que $$f(\rho )={\displaystyle \frac{f(b)–f(a)}{b–a}}$$

Entonces existe $\rho \in (a,b)$ tal que $$\overrightarrow{\alpha }(\rho )={\displaystyle \frac{\overrightarrow{\alpha }(b)–\overrightarrow{\alpha }(a)}{b–a}}$$

Si así fuera, entonces $$\Vert {\alpha }^{\prime }(\rho )\Vert ={\displaystyle \frac{\Vert \overrightarrow{\alpha }(b)–\overrightarrow{\alpha }(a)\Vert }{b–a}}$$

$\sum _{i=1}^n\Vert \alpha (t_i)–\alpha (t_{i-1})\Vert =\sum _1^n\Vert \alpha (t_i)–\alpha (t_{i-1})\Vert {\displaystyle \frac{t_i–t_{i-1}}{t_i–t_{i-1}}}$

$=\sum _{i=1}^n\Vert {\alpha }^{\prime }({\xi }_i)\Vert (t_i–t_{i-1})$

Por lo anterior definimos la longitud de arco desde $P$ hasta $Q$ como

$$\underset{a}{\overset{b}{\int }}\Vert {\alpha }^{\prime }(t)\Vert dt$$

CASO CIRCUNFERENCIA

Para $\omega =1.$

$x(t)=A\cos (t)+h$

$y(t)=A\sin (t)+k$

Derivando

$x^{\prime }(t)=–A\sin (t)⟹(x^{\prime }{)}^2(t)=A^2{\sin }^2(t)$

$y^{\prime }(t)=A\cos (t)⟹(y^{\prime }{)}^2(t)=A^2{\cos }^2(t)$

Sumando ambas igualdades

$(x^{\prime }{)}^2+(y^{\prime }{)}^2=A^2$ por lo que $\Vert {\alpha }^{\prime }(t)\Vert =A.$

Si $P=\alpha ({\theta }_0)$ y $Q=\alpha ({\theta }_1)$, entonces

$$\underset{{\theta }_0}{\overset{{\theta }_1}{\int }}Adt=A({\theta }_1–{\theta }_0)=\text{radio }\mathrm{\Delta }\theta$$

Una parametrización de una curva en coordenadas polares

Sea $r=f(\theta )$

Donde $\theta =\omega t$ y $r=f(\omega t)$, que en coordenadas polares es:

$x(t)=f(\omega t)\cos (\omega t)$

$y(t)=f(\omega t)\sin (\omega t)$

Si $\omega =1$ entonces $\overrightarrow{\alpha }(t)=(x(t),y(t))=x(t)\overrightarrow{e_1}+y(t)\overrightarrow{e_2}=r(t)\overrightarrow{\beta }(t)$, donde $\beta (t)=(\cos \theta (t),\sin \theta (t))$

En este caso, ¿cómo calculamos la velocidad?

$x^{\prime }(t)={\displaystyle \frac{d}{dt}}(f(\omega t)\cos (\omega t))=\omega f^{\prime }(\omega t)\cos (\omega t)–\sin (\omega t)f(\omega t)\omega$

$y^{\prime }(t)={\displaystyle \frac{d}{dt}}(f(\omega t)\sin (\omega t))=\omega f^{\prime }(\omega t)\sin (\omega t)+\cos (\omega t)f(\omega t)\omega$

Luego,

$(x^{\prime },y^{\prime })=(\omega f^{\prime }(\omega t)\cos (\omega t)–\sin (\omega t)f(\omega t)\omega ,\omega f^{\prime }(\omega t)\sin (\omega t)+\cos (\omega t)f(\omega t)\omega )$

$(x^{\prime },y^{\prime })=\omega f^{\prime }(\omega t)(\cos (\omega t),\sin (\omega t)+\omega f(\omega t)(-\sin (\omega t),\cos (\omega t)$

${\overrightarrow{\alpha }}^{\prime }(t)=r^{\prime }(t)\overrightarrow{\beta }(t)+r(t){\overrightarrow{\beta }}^{\prime }(t)$

$\beta$, ${\beta }^{\prime }$ son una base de ${\mathbb{R}}^2$ en la que podemos extresar ${\alpha }^{\prime }$.

$\overrightarrow{e_1}$, $\overrightarrow{e_2}$ son otra base de ${\mathbb{R}}^2$ en la que también podemos extresar ${\alpha }^{\prime }$.

Luego, ${\alpha }^{\prime }(t)=x^{\prime }(t)\overrightarrow{e_1}+y^{\prime }(t)\overrightarrow{e_2}=r^{\prime }(t)\overrightarrow{\beta }(t)+r(t){\overrightarrow{\beta }}^{\prime }(t)$