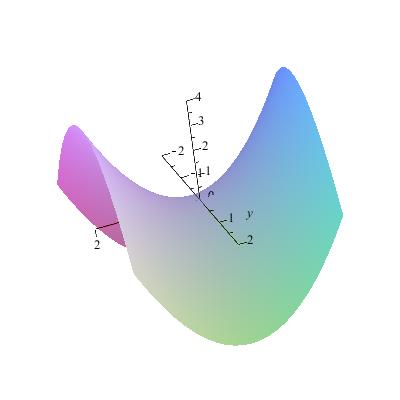Introducción
Los conjuntos de nivel proporcionan una representación visual de como la función toma ciertos valores en su dominio, mientras qu los límites nos permiten comprender el comportamiento de la función en puntos particulares o en el infinito. La relación entre ambos objetos puede verse como una descripción del comportamiento local y global de una función .
Funciones de
Definición 1 . Una función
Ejemplo. La función
Ejemplo. La función
Definición 2. El dominio de una función
Ejemplo. La función
Ejemplo La función
Definición 3.$ El rango de una función
Ejemplo. La función
Definición 4. La gráfica de una función
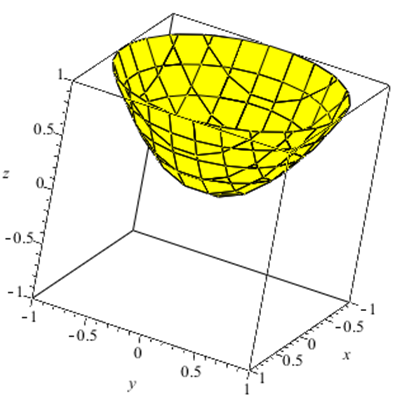
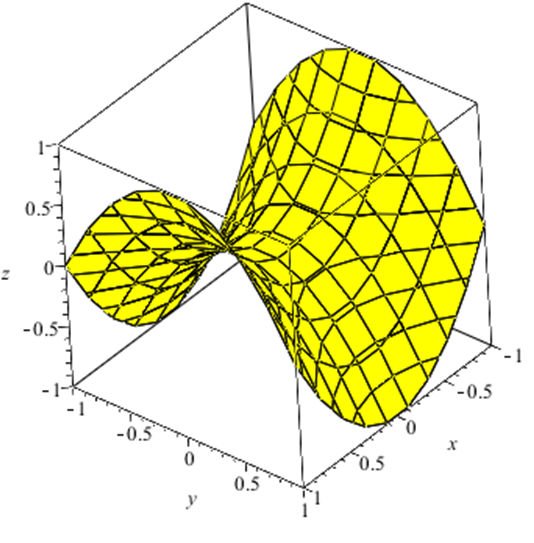
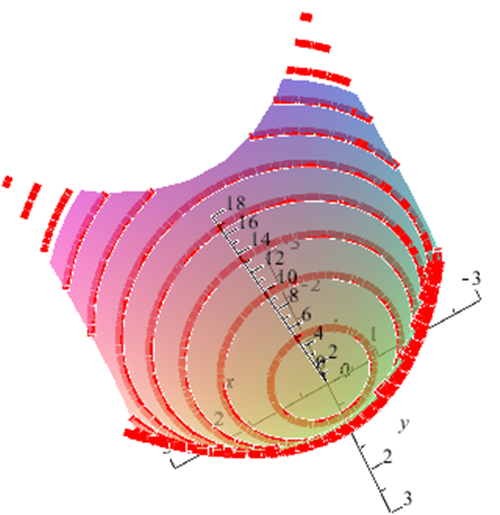
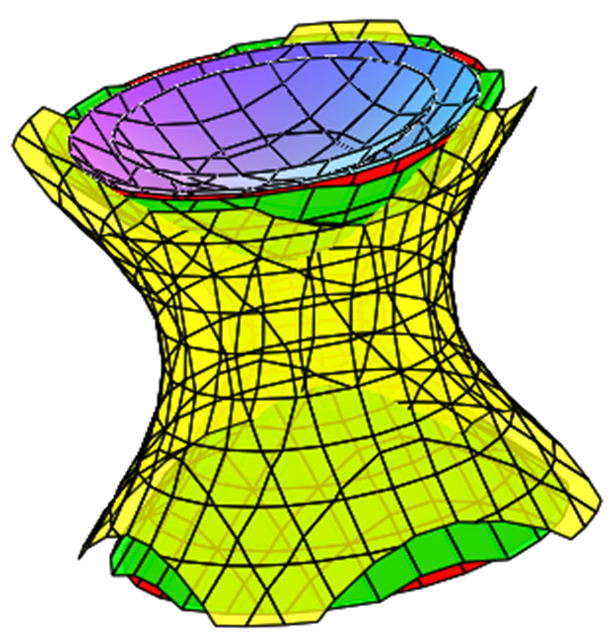
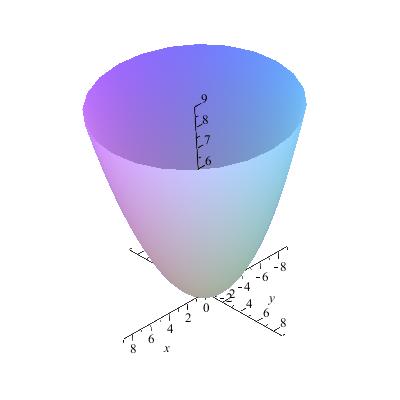
Ejemplo. La gráfica de la función
Ejemplo. La gráfica de la función
Conjuntos de Nivel
Definición 5. Sea
Ejemplo. Describir el conjunto de nivel de la función
geometricamente son circunferencias con centro el origen y radio
Ejemplo. Describir el conjunto de nivel de la función
Solución En este caso el conjnuto de nivel es
geometricamente son circunferencias con centro el origen y radio c
Ejemplo La función
Las curvas de nivel son: el vacio para
Ejemplo La función
Las curvas de nivel son: para
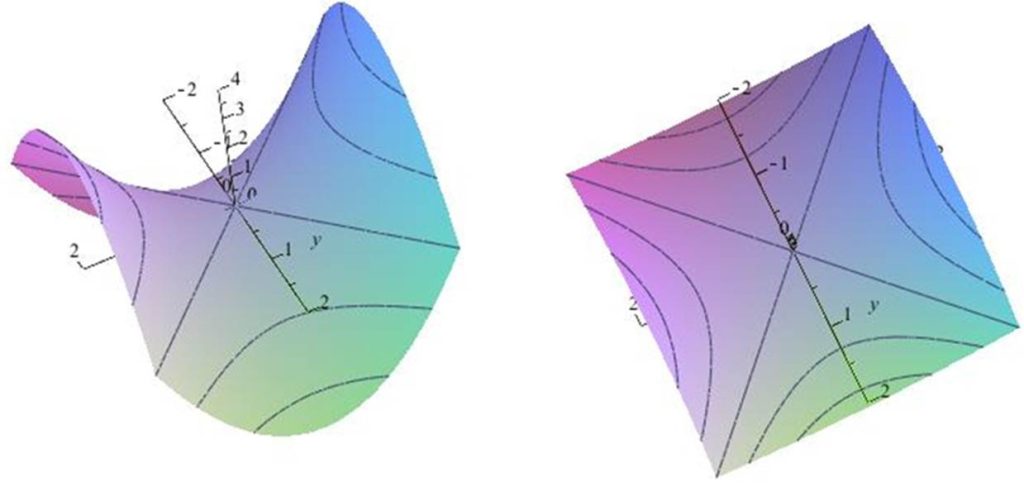
Ejemplo La función
Las superficies de nivel son: para
La función
Las superficies de nivel son: para
Límite de Funciones de
Sea
Observación: Es necesarío que
Usando la definición de límite, demostrar que:
Por demostrar, para todo
Demostración. Como
Más adelante
Relacionaremos el concepto de límite con el de derivada para funciones
Tarea Moral
1.- Esboza las curvas de nivel y gráficas de las siguientes funciones
a)
b)
2.- Describe el comportamiento conforme varia
a)
b)
3.- Traza la curva de nivel (en el plano
a)
b)
4.- Sea
5.- Sea
Enlaces
Calculadora para curvas de nivel de funciones de