Diferenciación de funciones
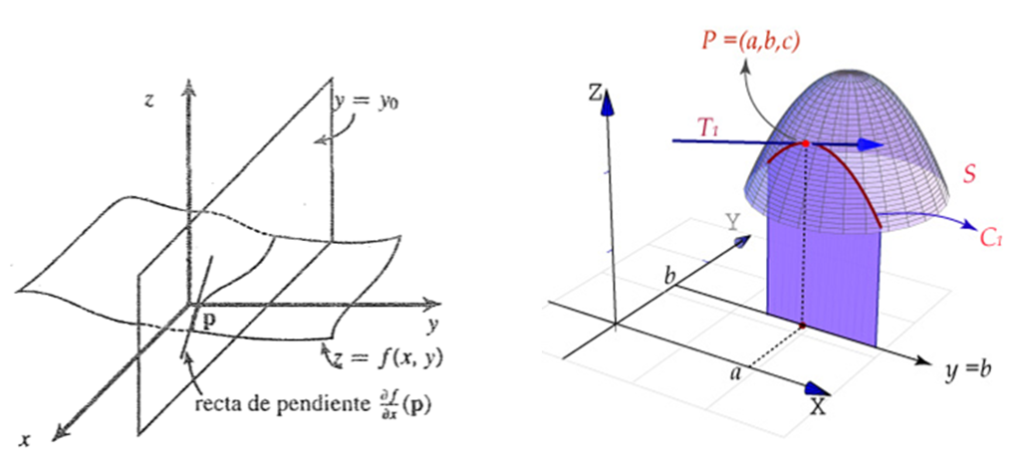
Sea
Sea
son:
Sea
Calcular
En este caso
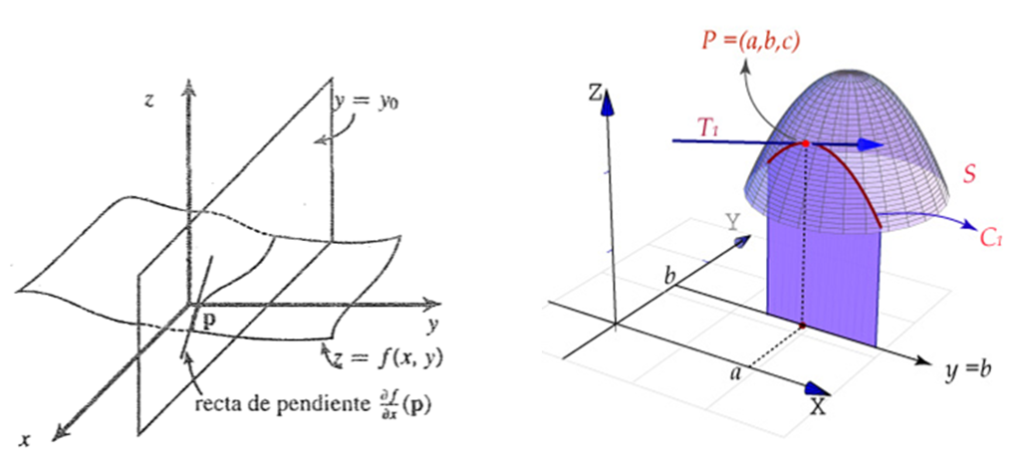
Ejemplo. Sea
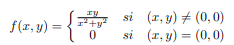
Calculemos
En este caso
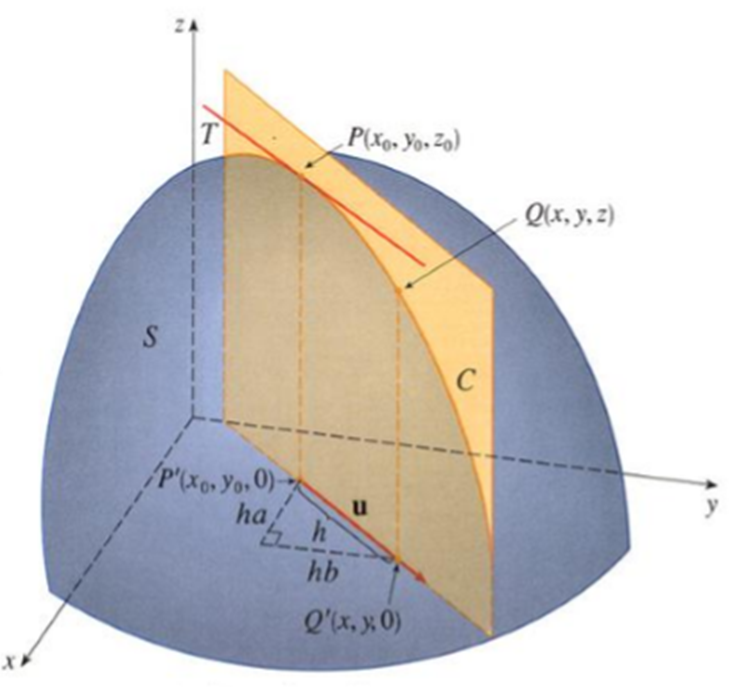
Derivada Direccional en un punto
Sea
la dirección del vector
Ejemplo. Sea
Notas: 1) La derivada direccional indica la variación de la función en la dirección de
2)Las derivadas parciales son derivadas direccionales respecto a los vectores de la base canonica.
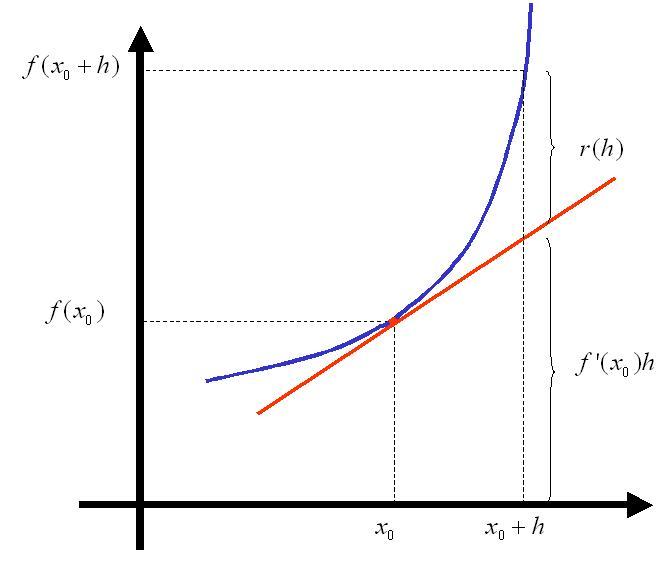
Diferenciabilidad
si
si
donde
Debería ocurrir
Definición. Sea
En la definición anterior si se toma
como
en consecuencia
En la definición anterior si se toma
como
en consecuencia
Definición. Sea
Más adelante
Tarea Moral
1.- Si
2.-Si
3.- Evaluar las derivadas parciales
a)
b)
4.-Mostrar que la siguiente función es diferenciable en cada punto de su dominio.
5.- Sea